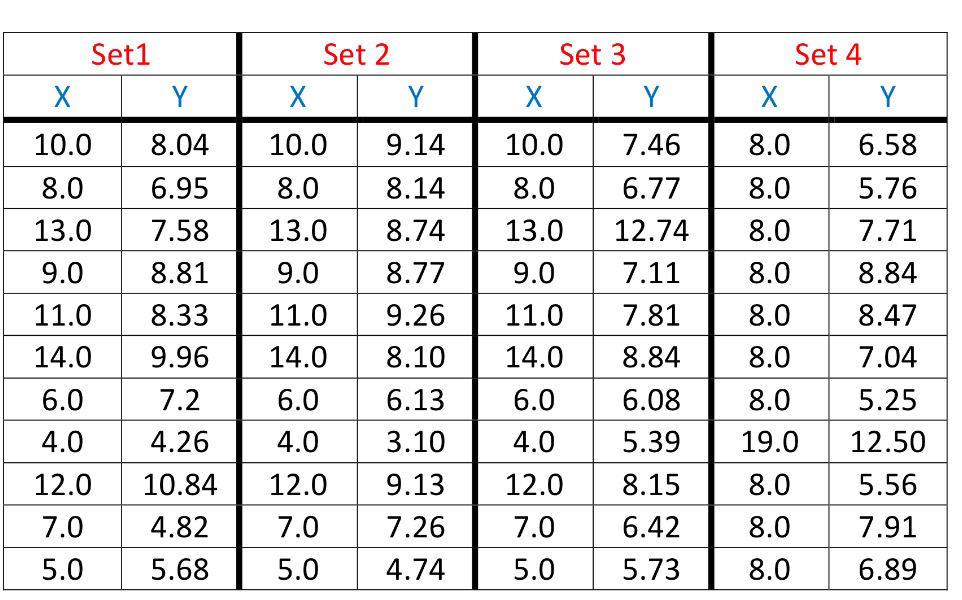
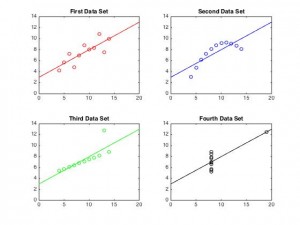
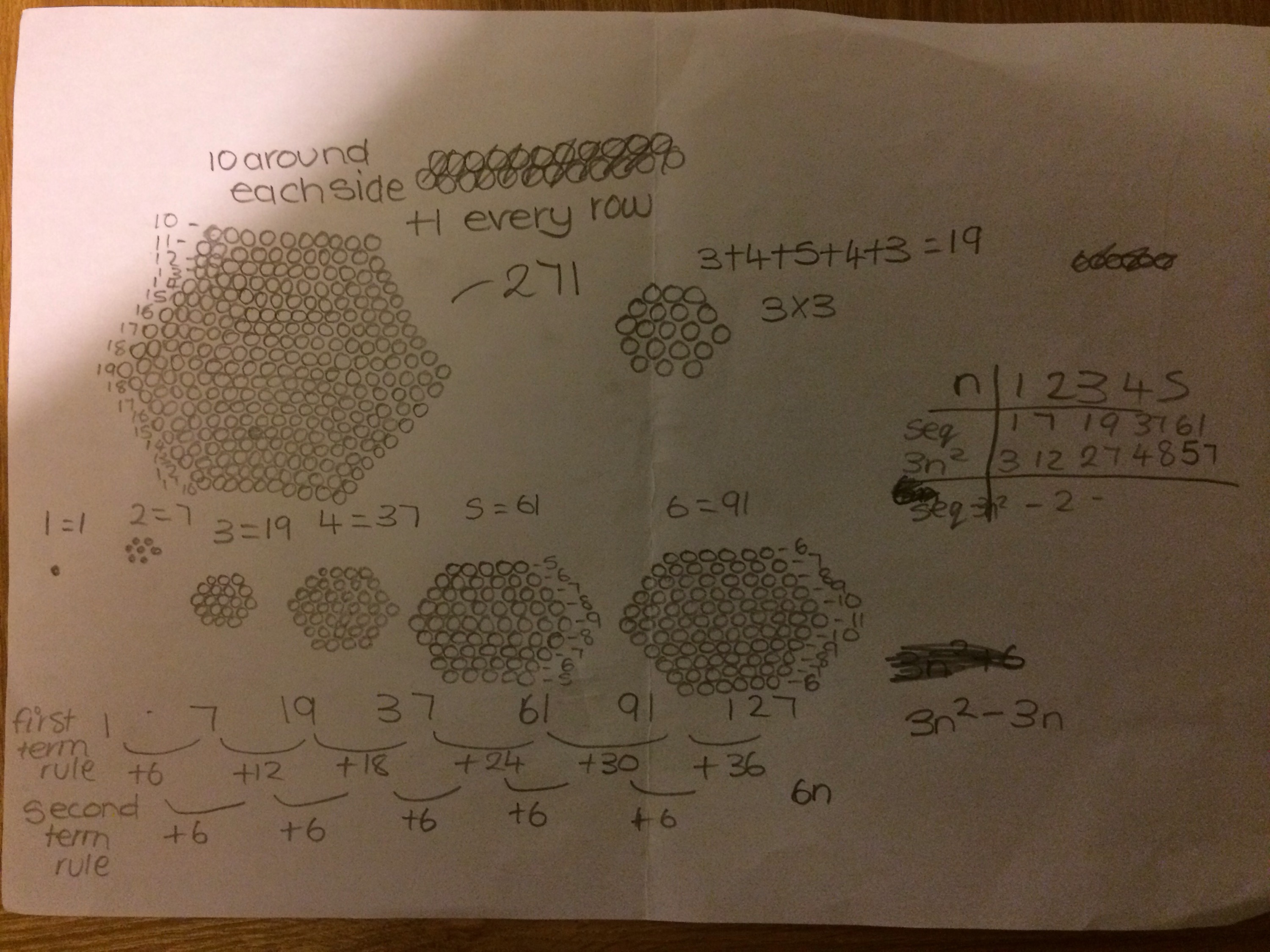
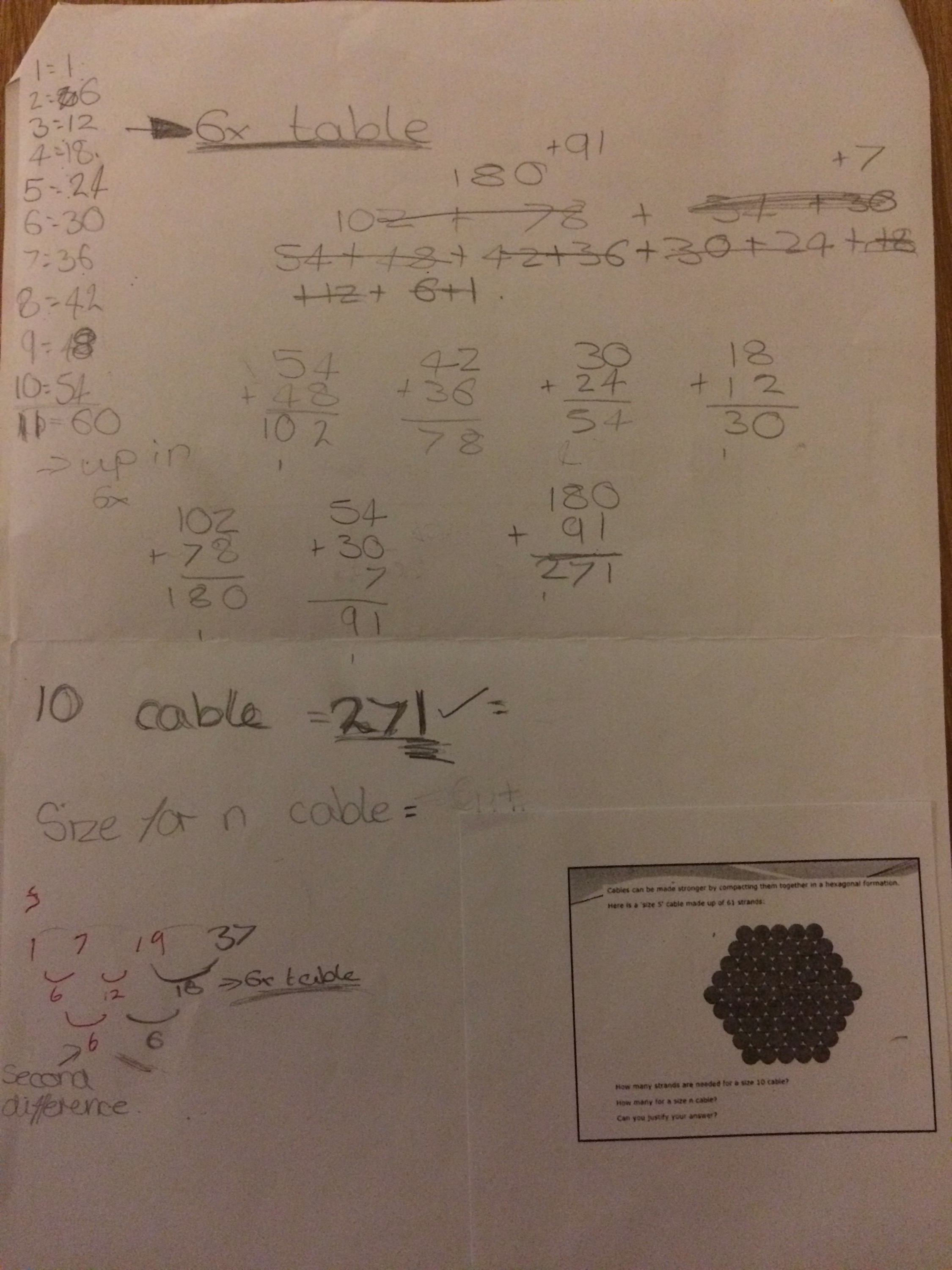
I recently read Manan’s post and learnt that in the States April is Mathematics Awareness Month. Manan had written about his mathematical journey so I thought I would do the same.
I remember always liking maths and number stuff, but apparently for the first couple of years at primary school I wasn’t seen as being good at it because I didn’t put in a lot of effort. I can remember having very boring SPMG (I think that was the acronym) work books, where you had to do things like “colour 12 balloons” – I was never good at colouring (I still can’t really colour in between the lines to be honest) and so I just didn’t do it. I probably wasn’t the best kid to teach, as far as I was concerned I could count and I didn’t need to colour things to prove it.
I loved primary school, and it was my Year 2 teacher who probably first made me “love” maths by giving me harder problems to solve than the rest of the class where I had to think more. This primary school also first introduced me to programming – first with logo and the big black “turtle” that moved on the floor and then with BBC BASIC on a BBC Micro and Acorn A3000 – though I didn’t know then that programming and maths would converge an become very important to me.
Whilst I was at school, I was also lucky enough to have lots of time with my grandmother, who had been a maths teacher (mainly at the Royal College for the Blind) who set me maths problems and sent me coded messages to crack which I always enjoyed. I can also remember doing maths to calm down when I was stressed or apparently hyperactive.
After having some good KS2 primary teachers I moved to secondary school, where I had a great teacher in Year 7, who again let me do work of my own choice most Friday lessons, including plenty of investigations. I still have a book on the history of Pi that he gave me when he left the school. People are often surprised that I didn’t enjoy the UKMT Junior Maths Challenges when I was at school – I didn’t really understand the benefit of them, and to me at the time they were puzzles that I didn’t really see the point of (I now think this is something that often needs addressing with KS3 students). I admit that I was probably seen as a bit of a geek at school – I once measureddhundred of blades of grass in an effort to determine if the grass around the school was all of the same type. I took my GCSE in Year 10 and then starter A Level Maths in Year 11. For me this was a good idea as it meant I could complete the A Level alongside starting Further Maths in Year 12 – this meant I avoided the problems of needing Year 13 content for Year 12 Further. My A level teacher was fantastic, always willing to help, or discuss things off the course. I appreciated how he always tried to show things from first principles, not just give us a result and then expect us to use it. At the end of Sixth Form I was pretty sure that following a maths degree I wanted to go and do a PhD – my uncle was (and still is) a lecturer in applied mathematics at Durham University – but in Pure mathematics, I was adament I didn’t want to do applied mathematics.
I went to study a 4 year Masters course at the University of Bath, in the end I chose a slightly odd mix of modules. I did a lot of Pure mathematics (group theory, number theory, measure theory etc) and lots of numerical methods, but very little fluids and mathematical modelling. This means I had a slightly odd background to choose (ironically) a PhD in applied mathematics. I had developed a love for the finite element method during some final year numerics modules. Such a simple idea but very powerful and infinitely less frustrating to analyse than finite difference methods with their taylor expansions! I applied to work with Paul Houston at the University of Nottingham, and following a brief meeting In the january of my final year was accepted to study under him and Andrew Cliffe.
I moved to Nottingham and initially I was meant to be working on anisotropic adaptivity for fluid problems, but about half way through my first year II changed topics and focussed on applying the Discontinuous Galerkin method to the Neutron Transport Equation – this turned out to be significantly harder than we anticipated. A lot of time in my PhD was taken up programming in Fortran. At some point I will probably write about this more….
To cut along story short, during my PhD I discovered that I felt more rewarded when I was teaching others – either tutoring undergraduate, lecturing postgraduates from other faculties in statistics or going into schools and running GCSE or A Level revision sessions or doing outreach events – than I did when I was doing my own research.
Following my PhD I applied for a School Direct place with the University of Nottingham.
That’s a fairly short run down on how I ended up in teaching. I’d love to hear the stories of other UK based teachers.