Following on from last months excellent collection of posts in the Carnival of Mathematics 126 from Stephen at cavmaths it is my turn to host this blog carnival.
The Carnival of Mathematics is a monthly blogging round up that is organised by The Aperiodical (check it out if for some reason you haven’t before) and has a different host each month.
It is tradition to start the post with some interesting facts about the number 127 so here goes….
- The most interesting fact about 127, I think, is that it is a Mersenne Prime. This means that 127 is a prime number of the form \(2^n-1\) with \(n\) here being 7. Since 7 is also a Mersenne prime 127 is known as a double Mersenne prime, that is, it is of the form \(2^{2^n-1} -1 \).
- 127 is a number that appears in the list of centred hexagonal numbers. Coincidentally I have written a post concerning these numbers when I discussed nRich’s Steel Cables problem here.
- Since 127 is a centred hexagonal number and prime it is Cuban prime.Namely it is a solution of the equation \( \frac{x^3-y^3}{x-y} \) where \(x=y+1\) and \(y>0\). This can be simplified so that Cuban primes are prime numbers of the form \(3y^2+3y+1\).
- 127 is a cyclic number. This means that the Euler totient of 127 and 127 are co-prime. (Clearly all primes are cyclic.)
- 127 is the 8th Motzkin number. For a particular value of \(n\) the \(n-\)th Motzkin number is the number of different ways of drawing non-intersecting chords between \(n\) points on a circle.
- 127 is a polite number as it can be written as \(127 = 63 + 64 \).
To start on the posts that make up this carnival I thought I would start with the following comic produced by Manan of MathMisery. This made me laugh a lot!
His blog is one that I routinely keep an eye on and I also enjoyed his recent post entitled Executive Education
Ganit Charcha submitted this really interesting article on Montgomery Modular Multiplication. I hadn’t heard of this algorithm before and I need to get round to coding it up soon – this article is very clear and written in a style ideal for being able to code it up.
Shecky R shared a short post looking at a curious finding (made without modern calculating machines) of Pierre de Fermat in his post Get A Life!
I enjoyed reading Tracy Herft’s post Unique Lesson… Polar Clocks. In it she describes a lesson she has developed for Year 7 students which she uses to link the topic of angles with fractions.
The mathematician Nira Chamberlain has shared his poster about the black heroes of mathematics. He has created this for Black History Month (which happens to be October).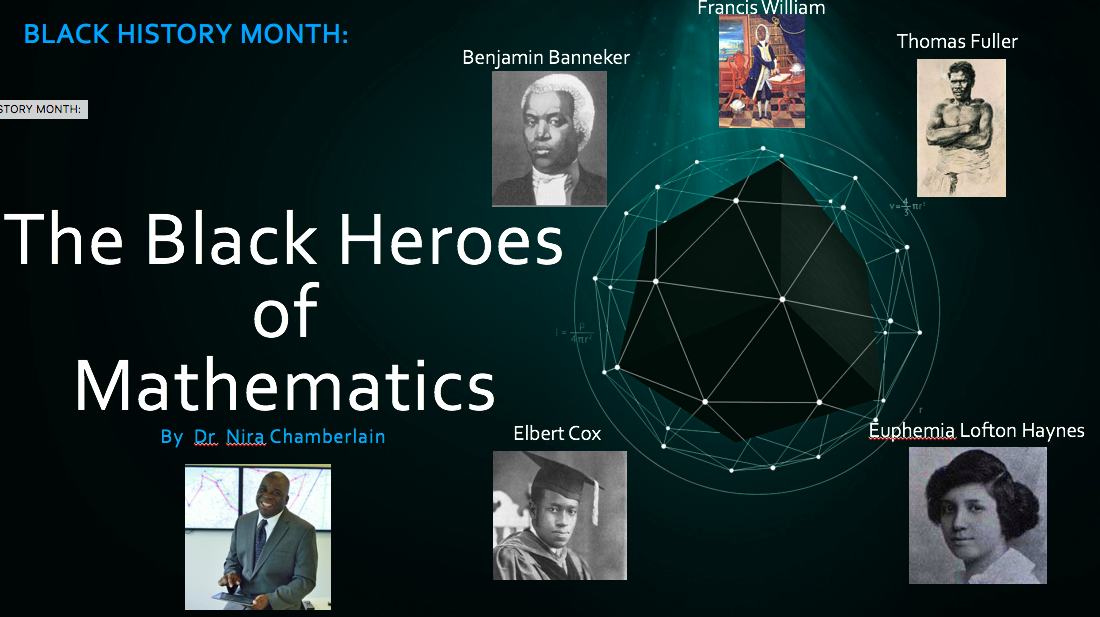
I’m ashamed to say that I hadn’t heard about any of these before!
This post by Evelyn Lamb (@evelynjlamb) concerning cutting letters of the alphabet featured a video by Katie Steckles of The Aperiodical. I love how Evelyn describes the “peculiar laziness” of mathematicians – it is certainly true!!
R.J. Lipton has this great accessible post “Frogs and Lily Pads and Discrepancy” discussing Terence Tao’s recently announced proof of Paul Erdös’ Discrepancy conjecture. If you fancy some more of the detailed maths take a read of Terence’s post announcing his papers (and the other related posts). Tao is pretty rare amongst professional mathematicians in that as well as publishing in academic journals he also discusses his research in an open blog.
Herminio L.A. submitted an interesting discussion of a calculation paradox in “Sabotage in the Stores”.
I had recently seen this video on Youtube and it was nice to learn that someone had submitted it for inclusion in this carnival (I would have myself if no one else had). The animations that go with some of the (many) patterns in Pascal’s triangle are fantastic.
Edmund Harriss has this post of beautiful animations of eigencurves of various matrices that are dependent on parameters in one entry. Edmund has also produced a colouring book with Alex Bellos titled “Snowflake, Seashell, Star” that taps in to the colouring craze at the moment. I can’t colour at all, but I am tempted to get this book, if only for the mathematical notes. He discussed the book here.
Christian Perfect (@christianp) shared this article by Lior Pachter. This article concerns something that I am very passionate about – the discussion of unsolved mathematical problems at school. Lior has taken each year in the American Common Core and selected an unsolved mathematical problem whose description is accessible to students of that age. I particularly like how each unsolved problem is accompanied by a starter problem that is accessible by the students. I think discussion of unsolved problems in school is very important as far too often students think that everything in mathematics is known. It’s sad that students s of school age don’t typically get exposed to current research or unsolved problems.
Diane has shared this problem from part of The Center of Maths’ Advanced Problem of the Week series. It’s quite a nice problem involving partial differentiation. This series provides a nice selection of problems to try, however some do require more area specific knowledge than others.
Peter Rowlett (@peterrowlett) recently wrote this fascinating post about Mathematical Myths. E.T. Bell did much to create a series of myths about mathematicians and some of these feature in this post. There are extensive links in this post so it may take a while to read.
During this month I enjoyed reading “The Nuts and Bolts of Writing Mathematics” by David Richeson and Cleve Moler’s blog (the first in a series of 4) on Dekker’s Algorithm. More recently Kris Boulton (@Kris_Boulton) had a great article about the importance of memorising times tables published in the TES.
Personally my most involved blog post in the last month was the write up of my NQT advice workshop at #msthsconf5.
This brings to an end this issue of the Carnival of Mathematics. The next edition is being hosted by Mike at Walking Randomly. If you haven’t before check out Mike’s blog, he regularly posts good articles about mathematical software.
One reply on “Carnival of Mathematics 127”
Thank you