In January I noticed that Steve Blades (@m4thsdotcom) was posting some interesting problems, many designed to stretch and promote thinking in students studying for their GCSEs.
I was particularly drawn to this question in the middle of January
Initially I thought this was harder than it was and was intending to use the information about the interior and exterior angles to form two simultaneous equations in \(x\) and \(n\) where \(n\) is the number of sides of the underlying polygon. However, there is of course a much simpler way if you use the fact that the interior and exterior angles of the polygon must add up to \(180^{\circ}\).
Using this fact you can obtain \(7x+1+28x+4 = 180\) and so \(35x = 175\) giving \(x = 5^{\circ}\). Hence the exterior angle of the polygon is \(7 \times 5 + 1 = 36^{\circ}\) meaning that the polygon has \(10\) sides and is a decagon.
I would be fascinated to see how students go about solving this question.
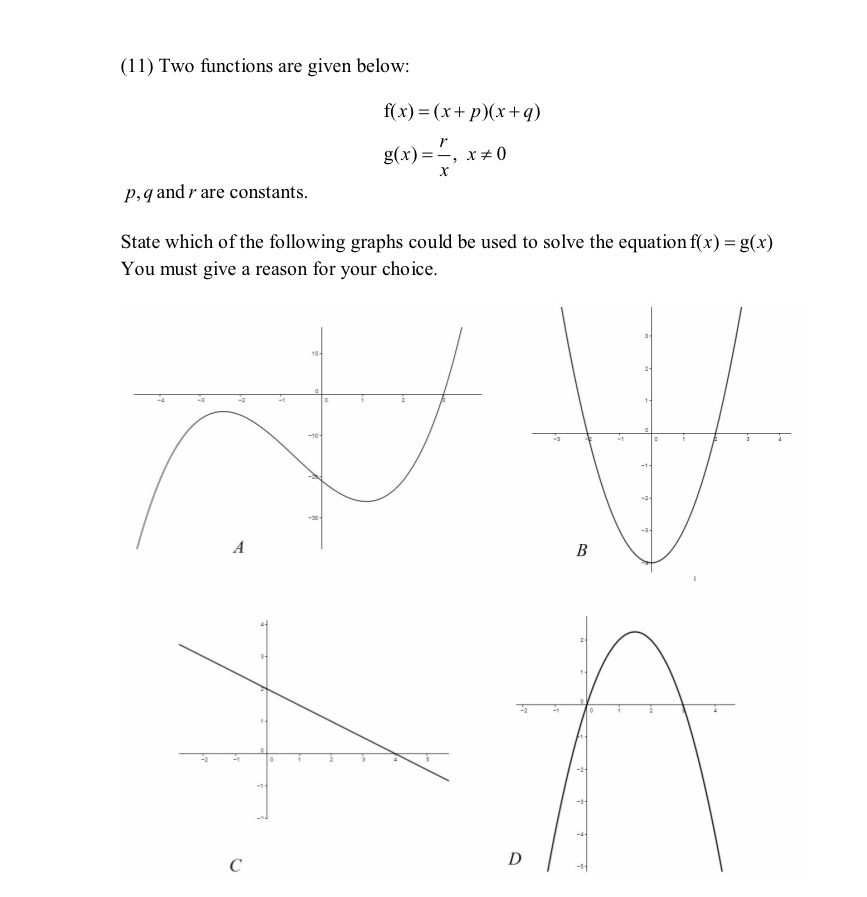
Steve has also collected a paper worth of challenging problems in a “Grade 9” paper available here. I particularly like this question about functions: